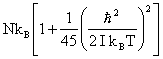Statistical Mechanics

PHYS 222

Dr. P. Misra

Fall 2002

Homework #8

Due: 11/8/02

1.

Use the Euler-Maclaurin formula ( f(x) is analytic for 0 < x < ∞ )

 = +-+-+…

to show that the rotational part of the heat capacity of a gas of heteronuclear diatomic molecules is given by:

 =

in the limit that .  I is the moment of inertia of one of the molecules.

2.

A system consists of N heteronuclear diatomic molecules embedded in a solid at temperature T.  Each molecule has a Hamiltonian:

H = +,

Where  is the angular momentum operator, I is a moment of inertia, φ is the azimuthal angle in spherical coordinates, and V_o is a constant (coming from solid-state effects).  Find the contribution of the molecules to the low temperature heat capacity of the system, keeping terms from the lowest four eigenstates.  Assume that , so that first order perturbation theory in V_o cos²φ is valid.

3

Calculate the magnetic susceptibility (in the limit of zero field) of a completely degenerate (i.e., T = 0) ideal Fermi gas.  Take the fermions to have spin one-half.